BUS 5413 Analysis Assignment 5
Statistical Refresher
The t-test for dependent groups has the same purpose as the one for independent groups. When each member of one group is paired or matched with a member of the other group, the data are called dependent, and the t-test for dependent groups should be used. This test is also known as the t-test for correlated data or the t-test for paired data.
Note that when a set of significance tests is presented in a table, those tests without a footnote indicating significance should be regarded as not significant.
Background Note
In the following excerpt, the data are paired in the sense that each participant responded to each item twice: The first time, each participant rated a characteristic of a bilingual friend; the second time, each participant rated the same characteristic of a monolingual friend. Thus, each pair of scores consists of the two responses of one participant.
Excerpt from the Research Article
A total of 46 bilingual (English and Spanish speaking) undergraduates (36 female, 10 male) participated. The average age was 20.6 years old, and the youngest student to participate was 18 years and the oldest 27 years.
Friendship Quality Scale (FQS). Participants answered 39 questions from the FQS (Bukowski et al., 1994) about their closest current bilingual and monolingual friendships. At the top, they were asked to choose their closest current bilingual and monolingual friendships and place their names at the top. Each item was only repeated once next to two scales (numbers 1 to 5 under the names of their closest friends). The FQS measures five aspects of friendship quality: closeness, security, help, companionship, and conflict. Means were created for each of these variables for the closest bilingual friend and monolingual friend.
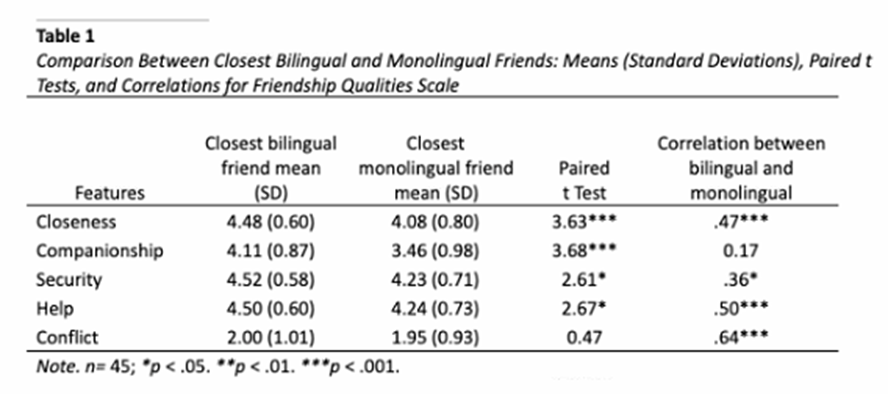
What is the value of the difference between the two means for “Closeness”?
| 0.4 |
| 4.48 |
| 4.08 |
Should the null hypothesis for the difference in your answer for Question 1 be rejected? Explain.
| Yes |
| No |
Is the difference in your answer for Question 1 statistically significant? Explain.
| Yes |
| No |
Should the null hypothesis for the difference in your answer for Question 4 be rejected? Explain.
| Yes |
| No |
Is the difference in your answer for Question 4 statistically significant? Explain.
| Yes |
| No |
The t-test is often used to test the significance of the difference between two means. It yields a value of p, which indicates the probability that chance or random sampling errors created the difference between the means. Most researchers declare a difference to be significant when p is equal to or less than .05. The lower the probability, the more significant the difference. Thus, a p of .01 is more significant than a p of .05. When a researcher declares a difference to be statistically significant, he or she is rejecting the null hypothesis. The lower the probability, the more confidence a researcher can have in rejecting the null hypothesis. (Holcomb, 2009)
Note that the absence of footnotes with probabilities in a table that contains a number of significance tests indicates that the comparison in question is not statistically significant.
Excerpt from the Research Article
The married sample comprised members of 100 heterosexual couples (54% were women and 46% were men) from a European university. The dating sample also consisted of members of 100 heterosexual couples (59% were women and 41% were men) from the same European university. All the married and dating couples were European. A researcher selected subjects in a haphazard fashion and asked them to complete a short survey. The mean age of the sample was 26.7 years (SD = 2.6).
The Affectionate Communication Index is a 19-item index that assesses the amount of verbal and nonverbal affectionate communication and support on a 7-point scale, with anchors of 1 = partners always engage in this type of affectionate activity to 7 = partners never engage in this type of activity. Thus, lower scores indicate more affectionate communication. Table 1 shows the results for the nonverbal activities.
Table 1
Dating and Married Individuals’ Reports of Nonverbal Affectionate Communication
| Item | n | M | SD | t | |
| Hold hands | Dating | 100 | 3.7 | 1.9 | 3.31 |
| Married | 100 | 2.9 | 1.7 | ||
| Kiss on the lips | Dating | 100 | 2.9 | 1.5 | 4.91 |
| Married | 100 | 2.0 | 1.1 | ||
| Kiss on the cheeks | Dating | 100 | 3.6 | 1.6 | 4.51 |
| Married | 100 | 2.7 | 1.3 | ||
| Give massages to each other | Dating | 100 | 4.2 | 1.8 | 5.01 |
| Married | 99 | 2.5 | 1.8 | ||
| Put arm around shoulder | Dating | 100 | 3.6 | 1.8 | 4.61 |
| Married | 92 | 2.5 | 1.5 | ||
| Hug each other | Dating | 100 | 2.5 | 1.6 | 2.0* |
| Married | 100 | 2.1 | 1.2 | ||
| Sit close to each other | Dating | 96 | 2.9 | 1.6 | 3.91 |
| Married | 100 | 2.0 | 1.6 | ||
| Look into each other’s eyes | Dating | 100 | 3.3 | 1.6 | 1 |
| Married | 100 | 3.0 | 1.8 | ||
| Wink at each other | Dating | 100 | 4.7 | 2.3 | 1.7 |
| Married | 96 | 4.2 | 1.9 |
*p < .05; 1p < .001. (Punyanunt-Carter, 2004)
Which group had a higher mean score for kissing on the lips (i.e., on average, which group reported less kissing on the lips)?
| a. Married Individuals |
| b. Dating individuals |
Is the difference between the two means for kissing on the lips statistically significant? If yes, at what probability level?
| a. No |
| b. Yes. At the p < .001 level |
| c. Yes. At the p < .05 level |
Should the null hypothesis be rejected for kissing on the lips? If yes, at what probability level?
Question options:
| a. No |
| b. Yes. At the p < .001 level |
| c. Yes. At the p < .05 level |
Is the difference between the two means for hugging each other statistically significant? If yes, at what probability level?
Question options:
| a. No |
| b. Yes. At the p < .001 level |
| c. Yes. At the p < .05 level |
Should the null hypothesis be rejected for hugging each other? If yes, at what probability level?
| a. No |
| b. Yes. At the p < .001 level |
| c. Yes. At the p < .05 level |
The differences are not statistically significant for which items?
| a. Sit close to each other and Look into each other’s eyes |
| b. Kiss on the lips and Give massages to each other |
| c. Look into each other’s eyes and Wink at each other |
| d. Put an arm around shoulder and Wink at each other |
Should the null hypothesis be rejected for “winking at each other”?
Question options:
| a. Yes | |
| b. No |
How many of the differences in Table 1 are statistically significant at the .05 level but not significant at the .001 level?
| a. One |
| b. Two |
| c. Three |
| d. Four |
The differences between the means for the following items are statistically significant. For which one is the significance at a higher level?
| a. The difference for hugging each other |
| b. The difference for sitting close to each other |
For which group can we reject the null hypothesis with greater confidence?
| a. The difference for hugging each other |
| b. The difference for holding hands |
For all items for which there are statistically significant differences, the means for dating couples are higher than the means for married couples. If you had planned this study, would you have hypothesized this result? Explain.
The table contains five types of statistics (n, M (µ), SD (σ), t, and p). Which three are descriptive statistics?
The table contains five types of statistics (n, M (µ), SD (σ), t, and p). Which two are inferential statistics?
The researcher states that she selected the individuals for this study “in a haphazard fashion.” What is your understanding of this term?
Probability and Statistical Significance
Ethnic Identity of Korean Americans
Statistics Refresher
Note that the lower the probability, the higher the level of statistical significance. For instance, p < .001 (1 in 1,000) is a higher level than p < .01 (1 in 100), and p < .01 is a higher level than p < .05 (5 in 100). Also, note that when probability levels are given as footnotes in a table (such as the table below), the absence of a footnote indicates that p > .05 (i.e., is greater than .05), and the associated difference between means is not statistically significant.
Excerpt from the Research Article
The sample consisted of 217 Korean American students selected from ethnically diverse high schools in the Los Angeles area.
Phinney’s 14-item Level of Ethnic Identity subscale was designed to assess the degree of ethnic identity among adolescents. Items such as “I am happy that I am a member of the group I belong to” and “I have a clear sense of my own ethnic background and what it means to me” (were included in the scale).
Phinney’s 6-item Attitudes Toward Other Groups subscale was designed to assess attitudes toward other groups. Respondents were asked to rate statements such as “I like meeting and getting to know people from ethnic groups other than my own.”
A five-item checklist was developed to assess perceived discrimination. Items asked respondents’ perception of society’s unfair treatment of their ethnic group and also the personal experience of unfair treatment. Typical items stated, “During your high school years, how often have you thought that your ethnic group is negatively stereotyped by mainstream people?” (Shrake& Rhee, 2004, p. 608).
What is the mean “Level of ethnic identity” for the total sample?
| a. 2.99 | |
| b. 3.00 | |
| c. 1.78 | |
| d. 3.01 |
Do the males or females have a higher mean on “Perceived discrimination”?
| a. Females | |
| b. Males |
Is the difference between the two means referred to in Question 2 statistically significant?
| a. Yes | |
| b. No |
Should the null hypothesis for the difference between the two means referred to in Question 2 be rejected?
| a. Yes | |
| b. No |
On “Other-group attitudes,” is the difference between the 13-15-year-olds and the 16-18-year­ olds statistically significant?
| a. Yes | |
| b. No |
On “Perceived discrimination,” is the difference between the 13-15-year-olds and the 16-18- year-olds statistically significant?
| a. Yes | |
| b. No |
Should the null hypothesis for the difference between the two means referred to in Question 6 be rejected?
| a. Yes | |
| b. No |
Four of the six values of t in Table I have asterisks that refer the reader to a footnote. Explain why two of the values do not have an asterisk.
The value of p for the t-test for the means of males and females for “Other-group attitudes” is p < .001. What does this mean?
| A. Less than 1 in 10 | |
| B. Less than 1 in 100 | |
| C. Less than 1 in 1,000 |
Which of the following differences between males and females is significant at a lower level?
| A. Other-group attitudes | |
| B. Perceived discrimination |
This research was conducted with Korean adolescents in the Los Angeles area. Would you be willing to generalize the results of this study to Korean adolescents who live in other regions of the country? Why? Why not?
Background Note
A researcher conducted two experiments on the effects of exercise and depression. The participants in the first study were adults who were above average in depression. The participants in the second study were 9 to 12-year-old students enrolled in an after-school exercise program. (Holcomb, 2009)
Excerpts from the Research Articles
Study l: Participants were recruited from a large corporation. Those desiring to begin an exercise program were designated exercise participants (the treatment group), and those not desiring to begin exercise at present were designated, control participants. The exercise group consisted of 26 participants and the control group (consisted) of 24.
Exercise participants were given a 10-week cardiovascular exercise program consisting of three 20 to 30-minute sessions per week.
The Profile of Mood States-Short Form Depression Scale requires users to respond to five one-word items (sad, gloomy, lonely, unworthy, discouraged) on a 5 point scale anchored by 0 = Not at All, and 4 = Extremely, to indicate “how you have been feeling during the past week including today” (1Annesi, 2005, p. 895)
Study 2: Twenty-six girls and 23 boys were recruited from a community-based, after-school program (to be in the treatment group). A control group (n = 41) from a similar after-school program that did not incorporate physical activity programming was added. The control group concentrated mostly on homework completion, reading, and tutoring.
The physical activity protocol (for the treatment group) included 3 days per week of cardiovascular activities in the form of non-competitive tasks and games, which alternated among low, medium, and high intensity over 12 weeks.
Depression and overall negative mood were assessed on the Profile of Mood States-Short Form. Included were scales of Depression (5 items) and Total Mood Disturbance (30 items).
At the beginning of Study 1, which group (Treatment or Control) had a higher mean depression score?
| Treatment | |
| Control |
At the end of Study 1, which group (Treatment or Control) had a higher mean depression score?
| Treatment | |
| Control |
In Study 1, which group had a lower mean depression score at Week 10 than at Week 1?
| Treatment | |
| Control |
In Study 1, the value of d for the Treatment group is negative. What does the negative indicate?
For the Treatment group in Study 1, the mean Week 10 depression score was how many standard deviation units different from the mean Week 1 depression score?
In Study 1, would it be appropriate to label either of the two effect sizes as large? If yes, for which group(s) is it large? Explain.
In Study 2, would it be appropriate to label any of the four effect sizes as large? If yes, for which group(s) is it large? Explain.
In Study 2, would it be appropriate to label any of the four effect sizes as moderate? If yes, for which group(s) is it moderate? Explain.
In Study 2, would it be appropriate to label any of the four effect sizes as trivial? If yes, for which group(s) is it trivial? Explain.
Examine the four means for the “Treatment” group in Table 2, which are reproduced below, along with the difference between Week 1 and Week 12, obtained by subtracting the mean at Week 12 from the mean at Week 1. The values of d are also shown.
Notice that the difference between the means is larger for “Total mood disturbance” than for “Depression.” Yet the effect size is larger for “Depression” than for “Total mood disturbance.” Does this make sense? Explain.
Note that the assignment to treatment and control conditions was not done at random. Is this a serious issue? Explain.
In light of the sample sizes, how much confidence do you have in the results of this study? Explain.
Does the fact that the researcher obtained similar results in two experiments using two very different types of participants increase your confidence in the results? Explain.
…Please click on the Icon below to purchase the FULL ANSWER at only $10




